Consider the rectangle drawn below,
Consider the properties of rectangle that the opposite sides are equal, and both the diagonals are also equal in length.
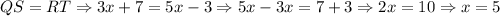
Thus, the value of 'x' is 5.
Substitute the value to obtain the diagonal QS as,
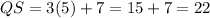
Similarly solve for the diagonal RT as,
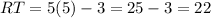
Already we knew that the diagonal will be equal with the length 22 units.