Question

the Firgure shows two triangles on a coordinate grid: Which set of transformations have been performed on triangle ABC to form triangle A'B'C'? A) Dilation by a scale factor of 1/3 followed by reflection about the x-axisB) Dilation by a scale factor of 3 followed by reflection about the x-axis C) Dilation by a scale factor of 1/3 followed by reflection about the y-axis D) Dilation by a scale factor of 3 followed by reflection about the y-axis

Answer 1

In transformations, the Pre-Image is the original figure and the Image is the figure transformated.

In this case you can identify that the Pre-Image is the triangle ABC and the Image is the triangle A'B'C'.

Notice that the vertices of ABC are:

`A(-3,3);B(0,0);C(-6,-3)`

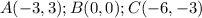
By definition, when the scale factor used in the dilation is between 0 and 1, the Image obtained is a reduction and, therefore, it is smaller than the Pre-Image. Since A'B'C' is smaller than ABC, then you can determine that ABC was dilated by this scale factor:

`sf=(1)/(3)`

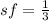
When a figure is reflected across the y-axis, the rule is:

`(x,y)\rightarrow\mleft(-x,y\mright)`

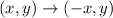
If you dilate ABC by the scale factor shown above, and then you reflect it across the y-axis, the coordinates of the Image will be:

`\begin{gathered} A\mleft(-3,3\mright)\rightarrow A^(\prime)(-((-3)/(3)),(3)/(3))\rightarrow A^(\prime)(1,1) \\ \\ B\mleft(0,0\mright)\rightarrow B^(\prime)\mleft(0,0\mright) \\ \\ C\mleft(-6,-3\mright)\rightarrow C^(\prime)(-((-6)/(3)),(-3)/(3))\rightarrow C^(\prime)(2,-1) \end{gathered}`

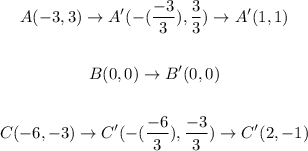
Notice that the coordinates of A'B'C' shown in the picture match with the vertices found above.

Therefore, the answer is: Option C.