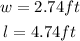We are given that the length a rectangular-shaped figure is 2 feet longer than its width. This can be written mathematically as:
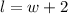
Where "l" is the length and "w" is the width. WE are also told that the area is 13 square feet. Since the area is the product of the length and the width this means the following:

From the previous equation we solve for the length by dividing both sides by its width:
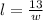
Now we replace this in the first equation:
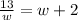
Now we multiply both sides by the width:

Subtracting 13 to both sides:
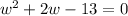
We get a quadratic equation. To solve this equation we will factor the equation by completing the square:
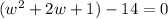
Factoring the parenthesis:
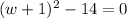
Now we add 14 to both sides:

Taking square root to both sides:
![w+1=\pm\sqrt[]{14}](https://img.qammunity.org/2023/formulas/mathematics/college/onrjckoms0uijruy3wursgsisvac8km55z.png)
Subtracting 1 to both sides:
![w=-1\pm\sqrt[]{14}](https://img.qammunity.org/2023/formulas/mathematics/college/euk99walclq2nex1be717ve53frnsw5k1m.png)
We take the positive value for the width, that is:
![\begin{gathered} w=-1+\sqrt[]{14} \\ w=2.74ft \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/7eia8xu72cvisgvj0bczr1okb74acbn6yk.png)
Now we replace this value of the width in the first equation:
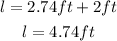
Therefore, the dimensions are: