Train A travels at a speed of 25 miles per hour south and train B travels at a speed of 20 mph east.
We can find the distance for each one of the trains by using the following formula:
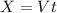
Where X is distance, V is velocity and t is time
Let's find the dinstance that A and B will travel in 6 hours

Train A travels 150 miles in 6 hours
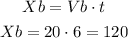
Train B travels 120 miles in 6 hours
Now, in order to determine how far they are from each other, we need to take into account their direction. From the picture we can see that their route describes a right triangle, so the distance between them is the hypotenuse of this right triangle I will draw...
if D is the distance that separates the two trains, D is given by the following formula
![D=\sqrt[]{150^2+120^2}](https://img.qammunity.org/2023/formulas/mathematics/college/rwl2374ry52fu51uupwh1n226ccpjhg98c.png)
Solving the equation we obtain...
![\begin{gathered} =\sqrt[]{22500+14400^{}} \\ =√(36900) \\ \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/be9320iog59teb9hvbgemde7k3r57j54ws.png)
Solving this square root by using prime factorization and laws of exponents:
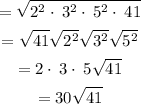
which is approximately the same as 192.09372...