Question

Please help me solve question 6 on my algebra homework

Answer 1

We have the following equation:

`y-5=2(x-2)`

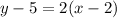
First, we leave the equation in the slope-intercept form.

`\begin{gathered} y=2x-4+5 \\ y=2x+1 \end{gathered}`

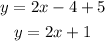
First, we leave the equation in the slope-intercept form.

Domain

The domain of a function is the set of the existence of itself, that is, the values for which the function is defined.

In this case, the solution is:

`-\inftyIn interval notation[tex](-\infty,\infty)`

Range

The range of the function is the set of all the values that the function takes in the existing interval of the domain.

In this case, the solution is:

`-\inftyIn interval notation[tex](-\infty,\infty)`

Zero

The zeros of a function are the points where the graph cuts the x-axis.

To find this, we equate the function to zero.

`\begin{gathered} 2x+1=0 \\ x=-(1)/(2)=-0.5 \end{gathered}`

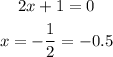
In this case, the zero is in -0.5.

Y-intercept

To find the y-axis intercept, we solve the equation when x=0.

`\begin{gathered} y=2\cdot0+1 \\ y=1 \end{gathered}`

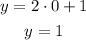
In conclusion, the y-axis intercept is in the coordinate (0,1)

Slope

Looking at the equation of the form y = mx+b we can easily tell what the slope is, remembering that "k" is the slope of the function.

`\begin{gathered} y=2x+1 \\ k=2 \end{gathered}`

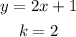
In conclusion, the slope is k=2

Type of slope

There are four different types of slopes: negative, zero, positive and undefined.

In this case, the slope is positive, because the angle of the slope is greater than zero and less than 90 degrees.

In conclusion, the slope is positive

f(3)

We will solve the function when x=3

`\begin{gathered} f(3)=2x+1 \\ f(3)=2\cdot3+1 \\ f(3)=6+1 \\ f(3)=7 \end{gathered}`

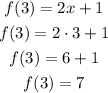
Value of x, where f(x)=7

We must equal the function to 7 and clear "x".

`\begin{gathered} 2x+1=7 \\ x=(7-1)/(2) \\ x=(6)/(2) \\ x=3 \end{gathered}`

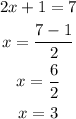
In conclusion, the value of "x" is x=3