Question

If you throw a 0.3 kg ball straight up with an initial speed of 32 m/s, how fast will it be moving when it’s 20 m above the release point? Round your answer to the nearest tenth and include the appropriate unit.

Answer 1

Given:

The mass of the ball, m=0.3 kg

The initial speed of the ball, u=32 m/s

The height, h=20 m

To find:

The speed of the ball when it is at a height of 20 m.

Step-by-step explanation:

The ball will be moving under the influence of acceleration due to gravity which is directed downwards.

From the equation of motion,

`v^2=u^2-2gh`

Where v is the final velocity of the ball and g is the acceleration due to gravity.

On substituting the known values,

`\begin{gathered} v^2=32^2-2*9.8*20 \\ \implies v=√(32^2-2*9.8*20) \\ =25.1\text{ m/s} \end{gathered}`

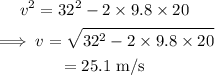
Final answer:

The velocity of the ball when it is 20 m above the releasing point is 25.1 m/s