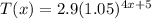Given:

Let's solve for T(x) which represents the approximate population in the region.
To find the approximate population in the region, apply the formula:
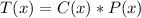
Thus, we have:
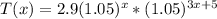
Let's solve the equation for T(x).
Thus, we have:

Therefore, the function that best describes the approximate population in the region is:
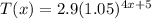
ANSWER:
C