Since we are dealing with a right triangle, we can use the following trigonometric identities
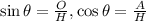
Where θ is an inner angle (different than 90°) of the triangle, O is the opposite side to θ, A is the adjacent side to θ, and H is the hypotenuse.
a) In our case,
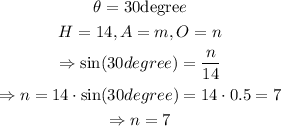
and
![\begin{gathered} \Rightarrow\cos (30degree)=(m)/(14) \\ \Rightarrow m=14(\cos (30degree))=14\cdot\frac{\sqrt[]{3}}{2}=7\sqrt[]{3} \\ \Rightarrow m=7\sqrt[]{3} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/xfiq9qif2b9cyoztrim1qqq9oqpj9uv9dv.png)
The answers are n=7 and m=7sqrt(3).
3) In a diagram, the problem states
Using the same trigonometric identities mentioned in part 1) (plus the tangent function), we get
![\begin{gathered} \sin (30degree)=(18)/(H),\tan (30degree)=(18)/(A) \\ \Rightarrow H=(18)/(\sin(30degree)),A=(18)/(\tan(30degree))=\frac{18}{\frac{1}{\sqrt[]{3}}}=18\sqrt[]{3} \\ \Rightarrow H=(18)/(0.5)=36,A=18\sqrt[]{3} \\ \Rightarrow H=36,A=18\sqrt[]{3} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/7oriymm9e2kotnwbwrntaqpsk7eb8a1v81.png)
The hypotenuse is equal to 36 ft, and the other leg is equal to 18sqrt(3) ft