1 ) According to the question, the temperature has been changing according to this function:
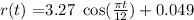
Where t is the number of hours after 6:00 am last Sunday
b) From 6 am to 1 pm last Sunday, the temperature in the park increased by
6 am to 1 pm = 7 hours, let's plug into that:
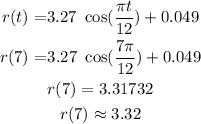
The rate of change in 7 hours was approximately 3.32 degrees per hour
c)
The temperature in the park at 6 am was 43.4 ºF. To find the temperature we must find the value for y, 1 pm Last Sunday. Let's plug the value already found: 1.68 for r.
Considering that according to question a, the temperature increased by 1.68º per hour. 6 am to 10 pm: 4 hours
c)
Since the question wants the temperature from 6 am to 1 pm, and it has been increasing by 3.32 degrees per hour, in 7 hours
7 x 3.32 =23.24º
43.4º+23.24=110.04ºF
d) On Friday, 5 days later there was
5 x 24 at 6am + 10 hours =120+10=130 hours
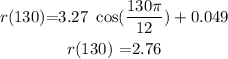
Starting from 43.4º F +2.76 =46.16ºF