We are given a triangle crossed by two parallel lines. The lines are parallel since their corresponding angles are the same. Therefore, from Thale's theorem we have the following relationship:
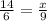
Now we solve for "x" by multiplying by 9 on both sides of the equation:
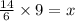
Solving the operations we get:
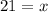
Therefore, x = 21