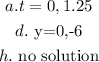1 Answer

Lance Hardwood · Answer 1 · 2024-01-03T02:06:24+0000

Given

The equations are given

a) 4t^2 - 5t = 0

d) (y + 6)(y - 6) = - (y + 6)^2

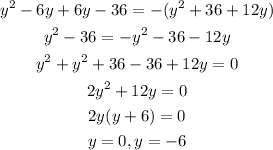
h) (u - 4)(u + 4) = (u + 2)(u - 2).

a. 4t^2 - 5t = 0

$\begin{gathered} t(4t-5)=0 \\ t=0,4t-5=0 \\ t=0,t=(5)/(4)=1.25 \end{gathered}$

d. (y + 6)(y - 6) = - (y + 6)^2

$\begin{gathered} y^2-6y+6y-36=-(y^2+36+12y) \\ y^2-36=-y^2-36-12y \\ y^2+y^2+36-36+12y=0 \\ 2y^2+12y=0 \\ 2y(y+6)=0 \\ y=0,y=-6 \end{gathered}$

h. (u - 4)(u + 4) = (u + 2)(u - 2).

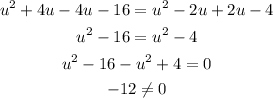
$\begin{gathered} u^2+4u-4u-16=u^2-2u+2u-4 \\ u^2-16=u^2-4 \\ u^2-16-u^2+4=0 \\ -12\\e0 \end{gathered}$

Answer

Hence the solution to the equations are

$\begin{gathered} a.t=0,1.25 \\ d.\text{ y=0,-6} \\ h.\text{ no solution} \end{gathered}$