Answer:
11 units
Step-by-step explanation:
Given that lines EB and DC are parallel, we use the proportional division theorem:
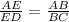
Substitute the given values:
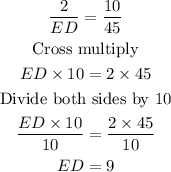
Next, find the length of AD:
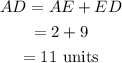
The length of AD is 11 units.
Alternate Method

So, we have that:
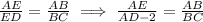
Substitute the given values:
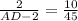
Cross multiply:
![undefined]()