Question

Sarah took the advertising department from her company on a round trip to meet with a potential client. Including Sarah a total of 18 people took the trip. She was able to purchase coach tickets for $170 and first class tickets for $1010. She used her total budget for airfare for the trip, which was $10620. How many first class tickets did she buy? How many coach tickets did she buy:

Answer 1

Step-by-step explanation

Let the number of people with coach tickets be x and the number of people with first class tickets be y. Since the trip goers contained a total of 18 people we will have;

`x+y=18`

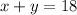
A coach ticket cost $170 dollars and the first class tickets cost $1010. Also, Sarah spent a total of $10620 to buy the tickets. This would give us;

`170x+1010y=10620`

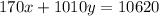
We will now solve the equation simultaneously.

`\begin{gathered} \begin{bmatrix}x+y=18\\ 170x+1010y=10620\end{bmatrix} \\ isolate\text{ for x in equation 1}\Rightarrow x=18-y \\ \mathrm{Substitute\:}x=18-y\text{ in equation 2} \\ 170\left(18-y\right)+1010y=10620 \\ 3060+840y=10620 \\ 840y=10620-3060 \\ 840y=7560 \\ y=(7560)/(840) \\ y=9 \\ \end{gathered}`

We will substiuite y =9 in x=18-y. Therefore;

`\begin{gathered} x=18-9=9 \\ x=9 \end{gathered}`

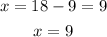
Answer: From the above, Sarah bought 9 coach tickets and 9 first-class tickets.