Question

there are 7 Red 3 blue and 5 green marbles in a bag what is the probability that the first three chosen will not be red?

Answer 1

Determine the total number of marble in the bag.

`\begin{gathered} n(T)=7+3+5 \\ =15 \end{gathered}`

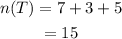
Determine the probability for first three marbles be red.

`\begin{gathered} P(R=3)=\frac{^7C_3^{}}{^(15)C_3} \\ =(35)/(455) \\ =(7)/(91) \end{gathered}`

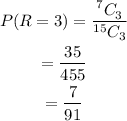
The probability for first three marble is not to be red is equal to one minus the probability for the first three marble be red.

`\begin{gathered} P(R\\e3)=1-P(R=3) \\ =1-(7)/(91) \\ =(91-7)/(91) \\ =(84)/(91) \\ =(12)/(13) \end{gathered}`

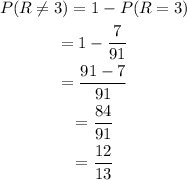
So answer is 12/13.