Question

Suppose you have a standard deck of 52 carts. What is the probability that if you select a card at random that it does not have a face value of 9? Round your answer to two decimals

Answer 1

A deck of cards has 13 face values: Ace, 2, 3, 4, 5, 6, 7, 8, 9, 10, Jack, Queen, and King. These face values are present for four different suits (Clubs, Diamonds, Hearts, and Spades) for a total of 52 cards.

An event's probability in an experiment with equally likely outcomes is defined by the following formula, where P (event) means the probability of the event occurring and is founding by dividing the number of outcomes in the event by the number of outcomes in the sample space:

`P(event)=\frac{Number\text{ }of\text{ outcomes in the event}}{Number\text{ of outcomes in the sample space}}`

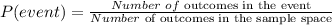
The probability of not getting the event is given to be:

`P^(\prime)(event)=1-\frac{Number\text{ }of\text{ outcomes in the event}}{Number\text{ of outcomes in the sample space}}`

Since there are 4 cards with a face value of 9, the probability of getting a 9 is:

`P(9)=(4)/(52)=(1)/(13)`

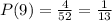
Therefore, the probability of not getting a 9 is given to be:

`\begin{gathered} P^(\prime)(9)=1-(1)/(13) \\ P^(\prime)(9)=(12)/(13) \end{gathered}`

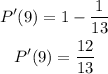
In two decimal places, the probability that the card picked does not have a face value of 9 is 0.92.