We know that:
- y varies directly as x
- y = 6 when x= 3
And we must find y when x = 9
To find it:
1. we must use that y varies directly as x

2. We must find k using that y = 6 when x = 3
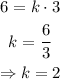
3. Finally, to find y when x = 9 we must replace x = 9 and k = 2 to solve it for y

ANSWER:
y = 18 when x = 9