Step 1 - Understanding how mole relates to temperature, pressure and volume
These variables are related according to the ideal gas equation:
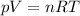
We can use this equation to predict the behavior as well as the quantity of a gas, given that the remaining variables are given.
Step 2 - Using the equation to solve the equation
According to the exercise:
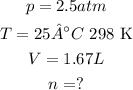
Setting the values in the equation:
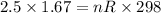
We need to make two observations now: the temperature must be in Kelvin in this equation for it to work. R stands for the universal gas constant, as has the value of 0.082 atm.L/mol.K.
Therefore:
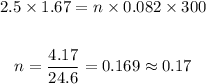
Answer: the amount of moles used is 0.17 moles.