Answer:

Step-by-step explanation:
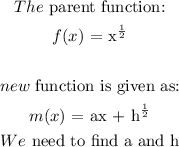
We need to translate the parent function in order to get the function for m(x).
The first thing we will do is to get the graph of the parent function. Then we will compare with the graph of m(x) to determine the transformation applied to it.
The parent graph starts at the origin (0, 0). But the graph of m(x) starts at -3. This means the graph was translated (moved) 3 units to the left.
When we move to the left, the value will be a positive number when writing the function
So our h = 3
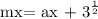
Next, is to find a:
The value of a could be less than 1, 1 or greater than 1. To determine the value, pick a point from the graph of m(x) given.
Using point (6, 6), we will substitute this value into the translated function to get a
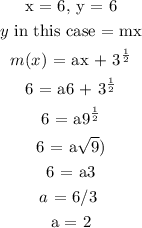
To ascertain we got the right function, I'll plot with the values of h and a we got and compare:

The graph above is the same as the given one in the question