Question

a) how much money will you have if you continue this investment for 10 years for 20 yearsb)write an exponential model that represents some amount in the account for any given year

Answer 1

Since the $5000 are the initial quantity when t = 0, we have the following exponential model:

`f(t)=5000(1.07)^t`

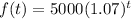
then, if we make t =0,1,2,3,4,5,6, we get the following table:

next, for the investment after 10 and 20 years, we have to make t = 10 and t = 20:

`\begin{gathered} t=10 \\ \Rightarrow f(10)=5000(1.07)^(10)=9835.76 \\ t=20 \\ \Rightarrow f(20)=5000(1.07)^(20)=19348.42 \end{gathered}`

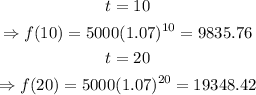
therefore, the total money of the investment after 10 years is $9835.76 and after 20 years is $19348.42