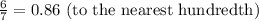The probability of an event is obtained as follows:
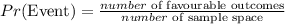
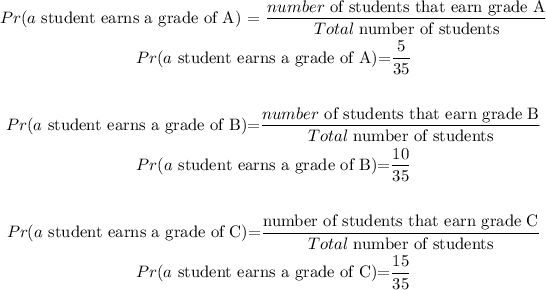
Therefore, the probability that a student earns a grade of A, B or C=
Pr(a student earns a grade of A) + Pr(a student earns a grade of B) + Pr(a student earns a grade of C).
This becomes;
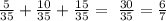
Hence, the probability that a student earns a grade of A, B or C is