Question

In a test run, a certain car accelerates uniformly from zero to 23.4 m/s in 2.90 s.(a) What is the magnitude of the car's acceleration? m/s2(b) How long does it take the car to change its speed from 11.7 m/s to 23.4 m/s? s

Answer 1

Given:

The initial velocity of the car is: u = 0 m/s

The final velocity of the car is: v = 23.4 m/s

The velocity of the car changes over the time t = 2.90 s

To find:

a) The magnitude of the car's acceleration

b) The time taken by a car to change its speed from 11.7 m/s to 23.4 m/s

Step-by-step explanation:

The acceleration of the object is defined as the rate of change of its velocity.

a)

Mathematically the acceleration of the car is given as:

`a=(v_-u)/(t)`

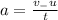
Here, a is the acceleration of the car.

Substituting the values in the above equation, we get:

`a=\frac{23.4\text{ m/s}-0\text{ m/s}}{2.90\text{ s}}=\frac{23.4\text{ m/s}}{2.90\text{ s}}=8.068\text{ m/s}^2\approx8.07\text{ m/s}^2`

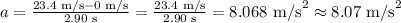
b)

The car accelerates uniformly, thus, the acceleration of the car when its velocity changes from zero to 23.4 m/s will remain the same.

Let w be the initial velocity. Thus,

w = 11.7 m/s

The time taken by the car to change its speed from 11.7 m/s to 23.4 m/s can be determined as:

`\begin{gathered} a=(u-w)/(t) \\ \\ t=(u-w)/(a) \\ \\ t=\frac{23.4\text{ m/s}-11.7\text{ m/s}}{8.07\text{ m/s}^2} \\ \\ t=1.45\text{ s} \end{gathered}`