To calculate the first term of the quadratic sequence given;
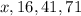
Take note that the nth term of a quadratic sequence is given by the formula;
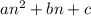
We shall use the terms provided to find the values of a, b and c, after which we shall use these to find the value of the first term.
Let us first of all confirm its a quadratic sequence by determining the first difference and the second difference.

We find the common difference for the first difference and this will serve as our 2nd difference.

We now divide the 2nd difference by 2 to get the value of a (in the equation for the nth term).

If a = 2.5, the the first term in the nth term is;
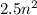
Next we substitute the numbers 1 to 4 into 2.5n^2 and we'll have;
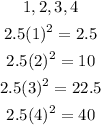
We now have a sequence which is;

And the differences are;
7.5, 12.5, 17.5
We shall take the nth term of this sequence of differences as follows;

With this result, we now have the values of b and c (to be used in the equation of the nth term).
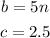
Where the equation is;
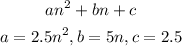
The first term becomes;
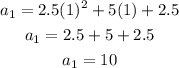
Withn this formula for the nth term you can go ahead and find any indicated term in this quadratic sequence.
ANSWER:
The first term is 10