Question

In a standard deck of cards there are 52 cards with 13 cards in each suit use this to find the following probabilities P(ace 2 or 3)P(spades or king)P(number card or black card)

Answer 1

In a standard deck of cards, there are four suites (club, diamond, heart and spade)

Each suite has 13 cards each. The 13 cards are ace, jack, queen, king, 2,3,4,5,6,7,8,9,10.

This means the number of each card is 4.

Number of aces = 4

Number of 2 = 4

Number of 3 = 4

Total number of cards = 52

Probability is calculated by ;

`\text{Probability = }\frac{number\text{ of required outcomes}}{nu\text{mber of possible outcomes}}`

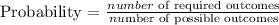
The P(ace, 2 or 3) will be;

`\begin{gathered} P(\text{ace, 2 or 3)= }\frac{totalno\text{ of ace, 2, 3}}{\text{total no of cards}} \\ P(\text{ace, 2 or 3)= }(4+4+4)/(52)=(12)/(52)=(3)/(13) \end{gathered}`

Therefore, the P(ace, 2 or 3) will be 3/13.

The P(spades or king) will be;

Number of spades = 4

Number of king = 4

`\begin{gathered} P(spadeorking)=\text{ }\frac{totalno\text{ of spades and kings}}{\text{total no of cards}} \\ P(spadeorking\text{)= }\frac{4+4}{\text{5}2}=(8)/(52)=(2)/(13) \end{gathered}`

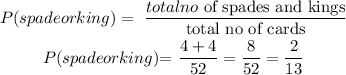
Therefore, the P(spades or king) will be 2/13.

P(number card or black card)

The number cards are 2,3,4,5,6,7,8,9,10. There are 9 number cards, and 4 in each suite.

This means we have 9 x 4 = 36 number cards in the deck of cards

Of the 36 numbered cards, half is black, half is red = 18 black numbered cards

Also, we have black and red cards in a deck of card

Number of Black cards = 26

Number of Red cards = 26

Therefore, the P(number card or black card) will be 11/13.