Question

Explain whether the following could be true: In a survey of 223students, 113 were taking algebra, 87 were taking biology, and 23were taking neither of the two subjects.

Answer 1

In a survey of 223 students, we have the following distribution;

`\begin{gathered} \text{Algebra}=113 \\ \text{Biology}=87 \\ \text{Neither}=23 \end{gathered}`

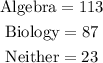
The distribution of students in the survey could be true because the number of students in each set when added up is equal to the total number surveyed.

This could mean that none of the sets had any elements common to one another, that is, there is no intersection of sets. Even if there were, the distribution of elements in the universal set is still equal to the number of elements in each set.

Therefore, this could be true.