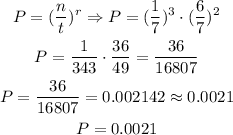Question

7.) If a letter is selected at random from the name MARILYN in five separate trials, what is the probability that the M is chosen exactly three times? Round your answer correctly to 4 decimal places. Your answer I

Answer 1

Let's begin by listing out the information given to us:

MARILYN (total, t) = 7 letters

At random implies every letter has an equal chance of being chosen

Number of trials (r) = 5 times

Number of "M" (n) = 1

Probability of choosing "M" once is given by:

`P=(n)/(t)=(1)/(7)`

what is the probability that the M is chosen exactly three times in five separate trials?

Of the 5 trials: 3 times "M" is picked, 2 times "other letters" are picked

`\begin{gathered} P=((n)/(t))^r\Rightarrow P=((1)/(7))^3\cdot((6)/(7))^2 \\ P=(1)/(343)\cdot(36)/(49)=(36)/(16807) \\ P=(36)/(16807)=0.002142\approx0.0021 \\ P=0.0021 \end{gathered}`