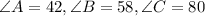Question

Solve the oblique triangle ABC a=25cm, b=32cm & c=37cm

Answer 1

Given

The sides of a triangle,

a=25cm, b=32cm & c=37cm.

To solve the oblique triangle ABC.

Step-by-step explanation:

It is given that,

The sides of a triangle,

a=25cm, b=32cm & c=37cm.

That implies,

By using cosine law,

`\begin{gathered} a^2=b^2+c^2-2bc\cos A \\ 25^2=32^2+37^2-2*32*37\cos A \\ 625=1024+1369-2368\cos A \\ 2368\cos A=1768 \\ \cos A=(1768)/(2368) \\ \cos A=0.7466 \\ A=\cos^(-1)(0.7466) \\ A=41.7\degree \\ A=42\degree \end{gathered}`

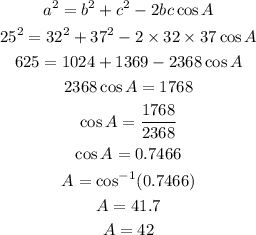
Also,

`\begin{gathered} (\sin A)/(a)=(\sin B)/(b) \\ (\sin41.7\degree)/(25)=(\sin B)/(32) \\ (0.6652)/(25)=(\sin B)/(32) \\ \sin B=(21.287)/(25) \\ \sin B=0.8515 \\ B=\sin^(-1)(0.8515) \\ B=58.37\degree \\ B=58\degree \end{gathered}`

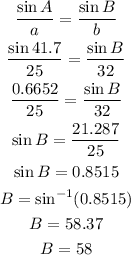
Therefore,

`\begin{gathered} \angle C=180-(\angle A+\angle B) \\ \angle C=180-(42+58) \\ \angle C=80\degree \end{gathered}`

Hence,

The answers are,

`\angle A=42\degree,\angle B=58\degree,\angle C=80\degree`