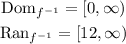Given the function f(x) defined as:
![f(x)=\sqrt[]{x-12}](https://img.qammunity.org/2023/formulas/mathematics/high-school/lcgctj5pnffrgrclh80mb8qirklczriifj.png)
(a)
To find the inverse of f(x), we express the function as:
![y=\sqrt[]{x-2}](https://img.qammunity.org/2023/formulas/mathematics/college/amrkxd1gku8t043bw0kdmy4e0wxe4occ5g.png)
Now, we take the square on both sides:
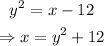
We change the notation:
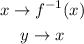
Then, the inverse function is:
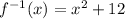
For x ≥ 0
(c)
The domain of f(x) are those values such that:
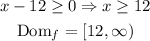
And the range is the set of all positive numbers (including 0):
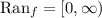
For the inverse, the domain of f(x) is its range, and the range of f(x) is its domain: