Hello there. To solve this question, we have to remember some properties about exponential functions and limits.
Given the options, we want to determine which of them could be an example of a function with domain:
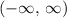
And range
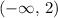
First, remember that exponential functions have the following form:
Each of the colored ones represent one type of exponential function:
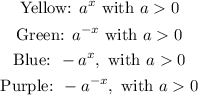
Of course, each of them have asymptotes at 0, but using translation properties of functions, we can determine a function with different asymptotes.
In this case, we're searching for a function with domain all over the real line, and it happens that all options satisfy this property, since all real numbers can be used in the function and give another real number as the input.
Then we have to search which of the functions satisfy the second condition.
Since we're searching for a function with range
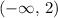
We want to find a function as the purple or blue ones, that start growing at -infinity and reaches an asymptote as x gets bigger.
Among the options, all of them have the minus sign in front which is a good indicator, next we have to determine their asymptotes.
We calculate the asymptotes by making

In this case, for each of the functions, we'll get:
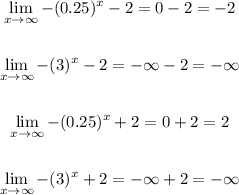
Therefore, the only options that seems to have an asymptote at y = 2 is the third option and correct answer.
Notice that
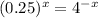
And that's why the limit is equal to zero as x goes to infinity (it gets infinitesimally small).