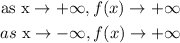End behavior
Answer1. The end behavior of a function describes the trend of the graph if we look to the right end of the x-axis (as x approaches + ∞) and to the left end of the x-axis (as x approaches - ∞).
For the polynomial
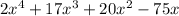
The end behaviors
The graph for the function is
Consider this graph of the polynomial function. Notice that as you move to the right on the x-axis the graph of the function goes up. We can describe the end behavior symbolically by writing
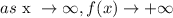
On the other end of the graph, as we move to the left along the x-axis, the graph of the function goes up, too. We can describe the end behavior symbolically by writing

Answer2: