Question

The function g is defined as follows for the domain given. g(x) = 2 - 2x , domain = \{- 1, 0, 1, 2\} Write the range of g using set notation . Then graph g

Answer 1

Step-by-step explanation:

The function is given below as

`g(x)=2-2x`

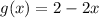
The set of domains is given below as

`\lbrace-1,0,1,2\rbrace`

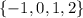
Step 1:

Put x=-1

`\begin{gathered} g(x)=2-2x \\ g(-1)=2-2(-1) \\ g(-1)=2+2 \\ g(-1)=4 \end{gathered}`

Step 2:

Put x=0

`\begin{gathered} g(x)=2-2x \\ g(0)=2-2(0) \\ g(0)=2 \end{gathered}`

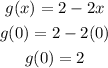
Step 3:

Put x=1

`\begin{gathered} g(x)=2-2x \\ g(1)=2-2(1) \\ g(1)=2-2 \\ g(1)=0 \end{gathered}`

Step 4:

Put x=2

`\begin{gathered} g(x)=2-2x \\ g(2)=2-2(2) \\ g(2)=2-4 \\ g(2)=-2 \end{gathered}`

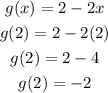
Hence,

The range of g(x) using set notation will be

`\Rightarrow\lbrace4,2,0,-2\rbrace`

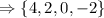
Hence,

The graph of g(x) is given below as