Question

You were given the polynomial f(x)=3x^3+13x^2+19x+k and you know that f(-2)=0find k

Answer 1

k = 10

Step-by-step explanation:

The initial polynomial is:

`f(x)=3x^3+13x^2+19x+k`

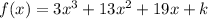
If f(-2) = 0, then when we replace x by -2, the result will be 0. It means that we can write the following equation:

`\begin{gathered} f(-2)=3(-2)^3+13(-2)^2+19(-2)+k=0 \\ 3(-2)^3+13(-2)^2+19(-2)+k=0 \end{gathered}`

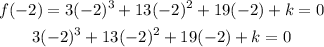
Therefore, we can solve for k as follows:

`\begin{gathered} 3(-8)+13(4)+19(-2)+k=0 \\ -24+52-38+k=0 \\ -10+k=0 \\ -10+k+10=0+10 \\ k=10 \end{gathered}`

So, the value of k is 10