Since the student is firing the cannonball horizontally this means the vertical component of the velocity is zero meters per second, that is 0m/s.
Now, to find how far the cannonball is from the base we have to remember that horizontally the velocity does not change in the motion; this means that horizontally we have a rectilinear motion with constant velocity. This motions are describe by the equation:

where x0 is the initial position, vx is the horizontal velocity and t is the time.
We know that in this case the initial position is zero, the horizontal velocity is 24 m/s and the time the cannonball is on the air is 3.22 seconds. Plugging this values we have:
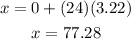
Therefore the ball lands 77.28 meters from the base.