Question

Find the average rate of change of f(x)=9x2-2 over the interval [0,7].

Answer 1

hello

to find the rate of change of f(x) over the interval [0 , 7], we'll have to substitute the values into the function

step 1

you'll substitute the intervals into the function so as to get f(0) and f(7)

`\begin{gathered} f(x)\text{ }=9x^2\text{ }-2 \\ \text{when f(0) =} \\ f(0)\text{ }=9(0)^2\text{ }-\text{ 2} \\ f(0)\text{ = 0 }-\text{ 2} \\ f(0)\text{ = }-2 \\ when\text{ f(7),} \\ f(7)\text{ }=9(7)^2\text{ }-\text{ 2} \\ f(7)\text{ = (9 }*\text{ 49) }-\text{ 2} \\ f(7)\text{ }=\text{ 441 }-\text{ 2} \\ \text{ }f(7)\text{ }=\text{ 439} \end{gathered}`

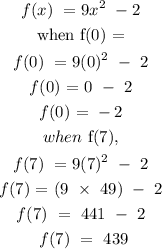
step 2

to find the average of the two functions, we'll add both of them and divide it by two

`\begin{gathered} \text{average rate }=\text{ }\frac{f(0)\text{ }+\text{ f(7)}}{2} \\ \text{average rate }=\text{ }\frac{\text{ }-2\text{ }+\text{ 429}}{2} \\ \text{average rate }=\text{ }(437)/(2) \\ \text{average rate }=\text{ 218.5} \end{gathered}`

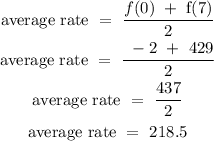
average rate of f(x) over the interval [0,7] is 437/2 or 218.5