Given
A local theater sold 187 tickets to a matinee play with a total revenue of $1,452.00 where they changed $11.00 for adult ticket and $6.00 for a child’s ticket.
Use the variables a and c to represent the number of adult tickets sold and the number of children’s tickets sold respectively.
To determine a system of equations that describes the solution.
1) Enter the equations below separated by a comma.
2) How many adult tickets were sold?
3) How many children’s tickets were sold?
Step-by-step explanation:
It is given that,
The total number of tickets sold is 187.
The total revenue is $1,452.00.
Since a and c represent the number of adult tickets sold and the number of children’s tickets sold respectively.
Then,

Solving (1) and (2) implies,
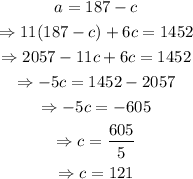
That implies,
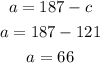
Therefore,
1) The equations are,
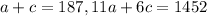
2) The number of adults ticket sold is 66.
3) The number of Children's ticket sold is 121.