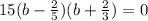A Quadratic equation can have the form:

Where "a" is the Leading coefficient.
In this case, you have the following Quadratic equation:
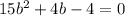
You can rewrite it as following:
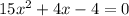
The steps to factorize it are shown below:
1. Use the Quadratic formula:
![x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}](https://img.qammunity.org/2023/formulas/mathematics/college/rxvf73usjbbwyik14knxdemoz21vfz2ufc.png)
2. You can see that:

3. Substitute values into the Quadratic formula and evaluate:
![\begin{gathered} x=\frac{-4\pm\sqrt[]{4^2-4(15)(-4)}}{2(15)} \\ \\ x_1=(2)/(5) \\ \\ x_2=-(2)/(3) \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/vllfautxnriq45ijfy9mq8n14adycs8ra7.png)
4. Substituting the variable "x" by the variable "b", you can write it in the following factor form:
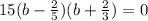
The answer is: