Question

The function R(t)=5600000e^−0.016t gives the number of farms in the United States t years since 1950. How many years after 1950 will the number of farms in the United States be 2,180,000?

Answer 1

given data:

The function

`R\mleft(t\mright)=5600000e^(-0.016t)`

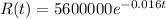
represents the number of farms in the United States t years since 1950.

to find How many years after 1950 will the number of farms in the United States be 2,180,000.

put t=59.

then,

`\begin{gathered} R(59)=5600000e^(-0.016(59)) \\ =5600000e^(-0.944) \\ =5600000(0.3890) \\ =2178783.29 \\ =2180000 \end{gathered}`

thus, after 59 the number of farms in the United States will be 2,180,000.