Question

Use interval notation to represent all values of x satisfying the given conditions

Answer 1

Given: The equation and inequalty below

`\begin{gathered} y=|3x-12|+1 \\ y<5 \end{gathered}`

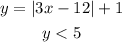
To Determine: The values of x satisfying the given conditions using interval notation

Solve the first equation

`y=|3x-12|+1`

The y-intercept, make x = 0

`\begin{gathered} y=\mathrm{\: }\mleft|3x-12\mright|+1 \\ y=|3(0)-12|+1 \\ y=|0-12|+1 \\ y=|-12|+1 \\ y=12+1=13 \\ T_{he\text{ coordinate of the y intercept is}} \\ (0,13) \end{gathered}`

The minimum point

`\begin{gathered} T_{he\text{ x coordinate of the minimum point}} \\ 3x-12=0 \\ 3x=12 \\ x=(12)/(3)=4 \\ T_{he\text{ y cordinate of the minimum point}} \\ y=|3x-12|+1 \\ y=|3(4)-12|+1 \\ y=|12-12|+1 \\ y=1 \\ T_{he\text{ coordinate of the minimum point is}}=(4,1) \end{gathered}`

Let us graph the two equation as shown below

From the graph above, the set of values of x that satisfies the equation and inequality can be seen from point A to point B.

Hence,

The solution is 2.667 < x < 5.333

Using interval notation we have (2.667, 5.333)