The speed of the ball bearing is given by the first derivative of the distance function.
Using the power rule in our function, we have
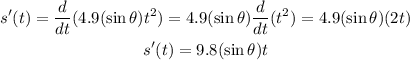
To complete the table we just have to evaluate the sine function on the given θ values.
![\begin{gathered} \theta=0\Rightarrow s^(\prime)(t)=9.8(\sin 0)t=9.8\cdot0\cdot t=0 \\ \theta=(\pi)/(4)\Rightarrow s^(\prime)(t)=9.8(\sin ((\pi)/(4)))t=9.8\cdot\frac{\sqrt[]{2}}{2}\cdot t=4.9\sqrt[]{2}t \\ \theta=(\pi)/(3)\Rightarrow s^(\prime)(t)=9.8(\sin ((\pi)/(3)))t=9.8\cdot\frac{\sqrt[]{3}}{2}\cdot t=4.9\sqrt[]{3}t \\ \theta=(\pi)/(2)\Rightarrow s^(\prime)(t)=9.8(\sin ((\pi)/(2)))t=9.8\cdot1\cdot t=9.8t \\ \theta=(2\pi)/(3)\Rightarrow s^(\prime)(t)=9.8(\sin ((2\pi)/(3)))t=9.8\cdot\frac{\sqrt[]{3}}{2}\cdot t=4.9\sqrt[]{3}t \\ \theta=(3\pi)/(4)\Rightarrow s^(\prime)(t)=9.8(\sin ((3\pi)/(4)))t=9.8\cdot\frac{\sqrt[]{2}}{2}\cdot t=4.9\sqrt[]{2}t \\ \theta=\pi\Rightarrow s^(\prime)(t)=9.8(\sin \pi)t=9.8\cdot0\cdot t=0 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/bbzpu2kftdv2lw5litqny25c8i6usiu9bu.png)
According to the table, the biggest value for the speed happens at θ = π/2.