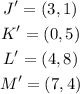Given the condition 90 degrees counterclockwise, we use rule below:
When rotating a point 90 degrees counterclockwise about the origin our point A(x,y) becomes A'(-y,x). In other words, switch x and y and make y negative.
Hence, To get J';
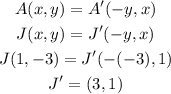
To get K':

To get L':
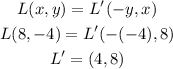
To get M':
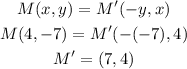
Therefore, the values of J',K',L' and M' are respectively given below;