We are asked to determine the perimeter of the given figure. The perimeter is found by adding the length of all the sides of the figure. Taking each square as a unit we divide the figure as follows:
we only need to determine the lengths of the exterior lines of the figure. For figure 1 we have a triangle that has base and height equal to 3 units, therefore, the length of the hypotenuse is:
![h=3\sqrt[]{2}](https://img.qammunity.org/2023/formulas/mathematics/college/pind659bwyytzgzc5ggso22aqmy43egcaz.png)
Figure 2 is an equal triangle as figure 1. For figure 3 we have 2 units on top and 2 units at the bottom, and for figure 4 we have a circle. The length of the arc of the circle is given by:
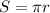
Where "r" is the radius. The radius of the circle is 3 units, therefore, the length is:
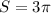
For this figure we must also add 2 units for the top and 2 units at the bottom. Therefore, the length of each side of the figure is:
![P=3\sqrt[]{2}+2+2+2+3\pi+2+2+2+3\sqrt[]{2}](https://img.qammunity.org/2023/formulas/mathematics/college/a5ijk4x9hj08uwl3p0qqfv44othvpkdtyr.png)
Solving the operations:
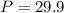
Therfore the perimeter is 29.9 units.
To determine the area we will add the areas of each figure. Figure 1 is a triangle and its area is:
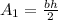
Replacing the values:
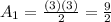
Since figure 2 is an equal triangle we have:
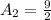
For figure 3 the area is the area of a square, that is:
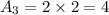
The area of figure 4 is the area of half a circle, that is:
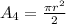
Replacing:
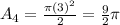
Adding each of the areas:
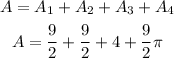
Solving the operations:
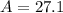
Therefore, the area is 27.1 square units.