Question

In the diagram, segment AB is tangent to Circle C at point B. Find the value of r (round to the nearest tenth as needed)

Answer 1

Since segment AB is tangent to the circle this means that angle ABC is a right angle, which in turns means that triangle ABC is a right triangle and then we can apply the pythagorean theorem:

`c^2=a^2+b^2`

where c is the hypotenuse, and a and b are the legs. In this case the hypotenuse has a length of r+8 and the legs have length r and 12. Plugging this in the theorem and solving for r we have:

`\begin{gathered} (r+8)\placeholder{⬚}^2=r^2+12^2 \\ r^2+16r+64=r^2+144 \\ r^2+16r-r^2=144-64 \\ 16r=80 \\ r=(80)/(16) \\ r=5 \end{gathered}`

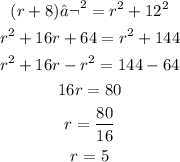
Therefore, the value of r is 5