ANSWER
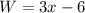
Step-by-step explanation
We have that the area of the rectangle is:
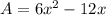
and the length is:
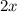
The area of a rectangle is given as:
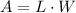
where L = length; W = width
This means that to find the width, we can divide the area by the length.
That is:
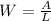
This implies that:
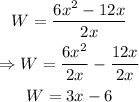
That is the width of the rectangle.