Question

You currently earn 800 per month but you are expecting your earnings to rise 70% per year. In five years what you expect to be earning?

Answer 1

To solve this problem we will use the following formula for compounded interest:

`A=A_0(1+r)^t,`

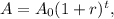
where A₀ is the initial amount, r is the interest rate as a decimal number, and t is the number of years.

Substituting A₀=800, r=0.7, and t=5 we get:

`A=800(1+0.7)^5.`

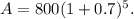
Simplifying the above result we get:

`A=800(1.7)^5=800\cdot14.19857=11358.856\approx11358.86.`

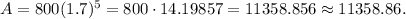
Answer:

In five years, you should be earning about $11360 per month, because your earnings rise 70% per year, which are added to the earnings of the previous month.