GIven:
The functions are
g(x) = 4x
h(x) = 2x-4.
The objective is to find the product (h*g)(x), subtraction (h-g)(x) and addition (h+g)(3) of the functions.
The value of product of the functions (h*g)(x) can be calculated as,
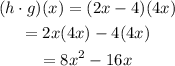
Now, the value of subtraction of the functions (h-g)(x) can be calculated as,
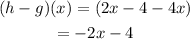
Then, the value of addition of the functions (h+g)(3) can be calculated as,
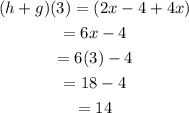
Hence, the results are,
(h*g)(x) = 8x²-16x
(h-g)(x) = -2x-4
(h+g)(3) = 14