Question

I solved this problems already. Just want to see if I got it correct.

Answer 1

Given the statement:

y varies directly as the cube of x.

When: x = 6, y = 36

Let's find the constant of variation and the variation equation.

Here, we are to solve using the direct variation equation:

y = kx

Where k is the constat of variation.

To find the constant of variation, k, substitute 6 for x and 36 for y.

Thus, we have:

y = kx

36 = 6k

Divide both sides of the equation by 6:

`\begin{gathered} (36)/(6)=(6k)/(6) \\ \\ 6=k \\ \\ k=6 \end{gathered}`

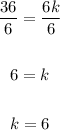
Therefore, the constant of variation is = 6.

The varitaion equation will be:

y = 6x

ANSWER:

• k = 6

• Variation equation,: ,y = 6x