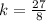Question

Can someone help me if you understand this type of stuff

Answer 1

The value of k is;

`k=(27)/(8)`

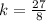
Step-by-step explanation:

Given the two similar Pyramids with surface area;

`\begin{gathered} A=29.16in^2 \\ B=2.56in^2 \end{gathered}`

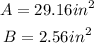
The scale factor is the ratio of their corresponding lengths. which will also be equal to the square root of the ratio of their corresponding Surface Area.

`k=\frac{\text{Length dimension of Pyramid A}}{\text{Length dimension of Pyramid B}}=\sqrt[]{\frac{\text{Surface area of Pyramid A}}{\text{Surface area of Pyramid B}}}`

Substituting the given surface areas;

`\begin{gathered} k=\sqrt[]{(29.16)/(2.56)} \\ k=\sqrt[]{(729)/(64)} \\ k=(27)/(8) \end{gathered}`

Therefore, the value of k is;

`k=(27)/(8)`