Question

Use coordinate methods (for example, distance formula, midpoint formula, or slope formula) todetermine whether this is a parallelogram. Explain your answer.8C = (5,7)7B10B=(-4,4)43N1-8 -7 -65 -4-3-2 -1 013587N-1D=(4,-1)-2-3-4A= (-6,-4)-5

Answer 1

We must prove that a set of points that forms a quadrilateral is a parallelogram or not. We will do it using the slope formula and the distance between the two points formula.

- The distance between two points is given by the following formula:

`d(A,B)=\sqrt[]{(x_B-x_A)^2+(y_B-y_A)^2_{}}`

- The slope m of a line a connect two points with coordinates (x_A,y_A) and (x_B,y_B) is the following:

`m(AB)=(y_B-y_A)/(x_B-x_A)`

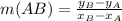
Now, from the graph, we see that the supposed parallelogram has the following sides: AD, DC, CB and BA.

To prove that this quadrilateral is a parallelogram we must prove:

1) First, the opposite sides have equal length.

2) Secondly, opposite sides have equal slopes.

1) We compute the length of the sides.

`\begin{gathered} AD=d(A,D)=\sqrt[]{(4+6)^2+(-1+4)^2}=\sqrt[]{109} \\ DC=d(D,C)=\sqrt[]{(5-4)^2+(7+1)^2}=\sqrt[]{65} \\ CB=d(C,B)=\sqrt[]{(-4-5)^2+(4-7)^2^{}}=\sqrt[]{90} \\ BA=d(B,A)=\sqrt[]{(-6+4)^2+(-4-4)^2}=\sqrt[]{68} \end{gathered}`

We see that opposite sides are not equal:

`\begin{gathered} AD\\e CB \\ DC\\e BA \end{gathered}`

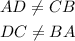
So we failed in proving the first requirement of the quadrilateral to be a parallelogram.

So we conclude that the set of points of the figure that forms a quadrilateral is not a parallelogram.