Question

On a number line, suppose the coordinate of A is 0 and AR=10. What are the possible coordinates of the midpoint of AR?The possible coordinates for midpoints of the segment are (Type integers or decimals.)

Answer 1

Given the line segment AR

The coordinate of A is 0.

AR=10

First, we determine the coordinate of R.

`\begin{gathered} |A-R|=10 \\ |0-R|=10 \\ |-R|=10 \\ R=10 \end{gathered}`

Therefore, the coordinate of R is 10(to the right of A

) or -10(to the left of A).

The midpoint of a number line is calculated using the formula below:

`\begin{gathered} \text{Midpoint}=(x_2+x_1)/(2) \\ \text{where }x_(2\; )and\; x_1are\text{ the endpoints of the line.} \end{gathered}`

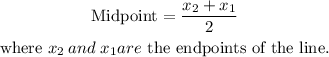
Therefore, the only possible coordinate for the midpoint of the segment AR is:

`\text{Midpoint}=(0+10)/(2)=5`

Therefore:

• If R is to the left of A, the midpoint of AR = -5

,

• If R is to the right of A, the midpoint of AR = 5

The possible coordinates for the midpoint of the segment AR are 5 and -5.