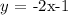Question

Which of the following is the equation of the line that contains the points (-1,1) and (3,-7)

Answer 1

The equation of the line passing through the two points is;

`y\text{ = -2x-1}`

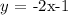
Here, we want to get the equation of the line that passes through the two given points

The general form of the equation is;

`\text{y = mx + b}`

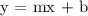
where m is the slope and b is the y-intercept

To get the slope, we use the slope equation

`\begin{gathered} m\text{ = }(y_2-y_1)/(x_2-x_1) \\ (x_1,y_1)\text{ = (-1,1)} \\ (x_2,y_2)\text{ = (3,-7)} \\ m\text{ = }(-7-1)/(3-(-1))\text{ = }(-8)/(4)\text{ = -2} \end{gathered}`

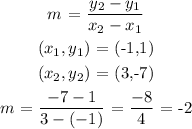
To get the y-intercept, we will need to substitute the coordinates of any of the points;

`\begin{gathered} y\text{ = -2x + b} \\ \text{substitute x = -1 and y = 1} \\ 1\text{ = -2(-1) + b} \\ 1\text{ = 2 + b} \\ b\text{ = 1-2 = -1} \\ \\ \end{gathered}`

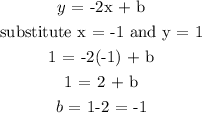
The equation of the line is thus;

`y\text{ = -2x-1}`