Question

A flatbed truck travels with a constant speed v = 28 m/s in a circle of radius R = 112 meters in a flat parking lot. A box which rests in the truck bed is held in place only by friction.(a) What is the centripetal acceleration of the box?(b) What is the minimum coefficient of static friction necessary to keep the box from sliding in the truck bed?[Use g = 10 m/s^2]

Answer 1

Given that the speed of the truck is, v = 28 m/s and the radius of the circle is, R = 112 m.

We have to find centripetal acceleration.

The formula of centripetal acceleration is

`a_c=(v^2)/(r)_{}`

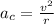
Substituting the values in the above formula, we get

`\begin{gathered} a_c=((28)^2)/(112) \\ =7m/s^2 \end{gathered}`

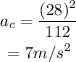
Thus, the centripetal acceleration is 7 m/s^2.

(b) Condition to find the minimum coefficient of static friction is

`F_c=F_f`

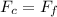
Here, F_c is centripetal force anf F_f is the frictional force.

Also,

`F_c=ma_c`

Here, m is the mass of the block.

The frictional force is given by the formula,

`F_f=\mu mg`

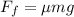
Here, mu is the coefficient of friction, g is the acceleration due to gravity where, g = 10 m/s^2

Equating centripetal force and frictional force, we get

`\begin{gathered} ma_c=\mu mg \\ \mu=(a_c)/(g) \end{gathered}`

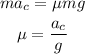
Substituting the values, we get

`\begin{gathered} \mu=(7)/(10) \\ =0.7 \end{gathered}`

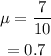
Thus, the minimum coefficient of static friction is 0.7